Detailed Float in CPM

Calculating Float in the Critical Path Method
Total Float and Free Float are two terms used in the “Critical Path Method” (CPM). There are many people, even project managers, who still believe that both these terms are just synonymous to each other. In fact Total Float and Free Float are two different concepts:
- Total Float in CPM is the amount of time an activity can be delayed without delaying the project finish date.
- Free Float in CPM is the amount of time an activity can be delayed without delaying the Early Start of its successor activity.
Total Float in CPM
Total Float, also known as Slack, and the value of Total Float is zero on a critical path. There are two methods to calculate the Slack. You can subtract the duration of the non-critical path from the critical path. You can calculate the slack also by subtracting the Early Start from the Late Start (LS-ES) or by subtracting the Early Finish from the Late Finish (LF-EF).
Free Float in CPM
For calculating the Free Float, you are required to subtract the Early Finish of the activity from the Early Start of the next activity (ES of next activity – EF of current activity). You need to modify this formula slightly if you are not considering 0 as your project’s start date.
If you are considering to have the project start date as 0, then the formula to find Free Float is ES (Early Start) of next activity – EF (Early Finish) of current activity. On the other hand, if you count from 1 (consider 1 as your projects starting day), then you need to subtract 1 from the above formula. So, the formula becomes ES of next activity – EF of current activity – 1.
Float in CPM Calculations
You should know that the Early Start and the Early Finish are marked at the top corners of the box and the Late Start and the Late Finish are marked at the bottom corners of the box as shown below.
- ES – Early Start
- EF – Early Finish
- LS – Late Start
- LF – Late Finish
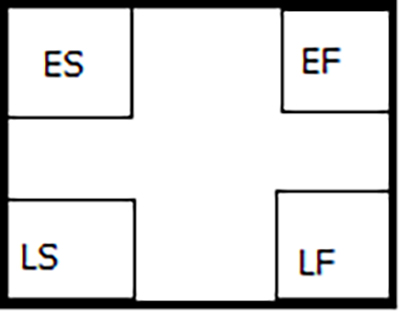
The duration is marked inside the box. Let’s consider the following diagram.
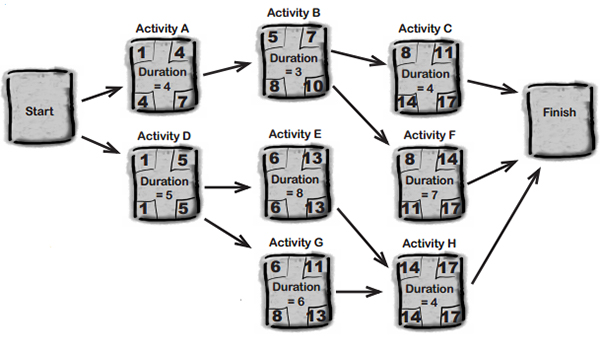
You can see four different paths from start to finish.
- A-B-C
- A-B-F
- D-E-H
- D-G-H
If you calculate the duration for each path you will get the values as
- A-B-C 🠺 4+3+4 = 11 Days
- A-B-F 🠺 4+3+7 = 14 Days
- D-E-H 🠺 5+8+4 = 17 Days
- D-G-H 🠺5+6+4 = 15 Days
It is clear that D-E-H is one the critical path because it has the longest duration of 17 days.
Calculate Total Float
First, let’s calculate the total float by subtracting the duration of the non-critical path from the critical path. In our case,
Total Float = (duration of path D-E-H) – (duration of path D-G-H)
= 17 – 15 = 2 Days
Thus, the Total Float is 2 days. Let’s confirm this with second method.
Total Float for activity G is (LF-EF) or (LS-ES)
= (13-11) or (8-6) = 2 Days
Please note that Total Float is zero on the critical path. Next, let’s calculate the Free Float. It is obvious that the activities on the critical path cannot have a free float. Let’s calculate the Free Float for activity G.
Free Float Activity G
Free Float for activity G is ES of next activity – EF of current activity -1
= (ES of activity H) – (EF of activity G) – 1
= 14 – 11 – 1 = 2 Days
Free Float of activity G is 2 days.
Summary – Float in CPM
Total Float and Free Float are two different concepts in the Critical Path Method. Total Float deals with the amount of time an activity can be delayed without delaying the project finish date. While, Free Float deals with the amount of time an activity can be delayed without delaying the Early Start of its successor activity.
